
Chaos theory, catastrophe theory, and complexity are all examples of nonlinear dynamical
systems theory or nonlinear science. The central idea is that systems change over time in many
possible patterns that we can analyze and evaluate. Events that seem to be random might not be
random at all. The specific concepts of nonlinear change, which have mathematical origins, are
explained here along with their evolving applications in psychology, biomedical sciences, organizational
behavior, and economics.
See News

Basic Dynamics
Attractors are spatial structures where,
if an object enters it, it does not leave.
Repellors and saddles also fall into this
family of dynamics.
Bifurcations are patterns of instability
that divide a dynamical field into parts
containing different dynamics taking
place inside.
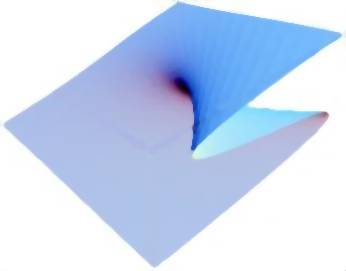
Catastrophe Theory
Catastrophes are sudden changes of
events. They do not necessarily imply
disaster. Theory describes a set of 7
models, although the cusp, one of the
simplest is the most commonly used.
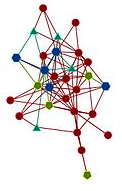
Networks
Networks represent communication
patterns among neurons, people, and
larger sociotechnical entities. Many aspects of complex dynamics contribute
to their formation and behavior. It's a
small world out there!
Applications
We have organized them into five broad categories for your convenience. Start anywhere!
Psychology
Biomedical Sciences
Organizational Behavior
Economics
Education
Biomedical Sciences
Organizational Behavior
Economics
Education
Conferences
SCTPLS sponsors the longest-
running annual conference
in its topic area. All nonlinear
scientists are invited. Explorers
meet world-class experts in a
convivial supportive environment.
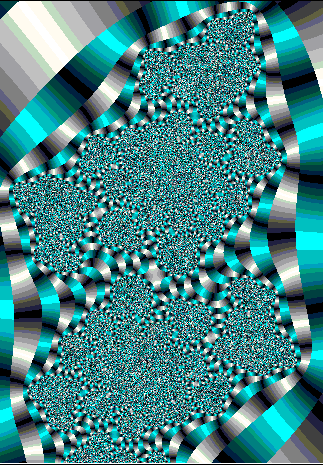
Chaos and Fractals
Many seemingly random events, which are
actually chaotic, are predictable with simple
equations. They are sensitive to small
differences in their initial values, however,
producing what is commonly known as
the butterfly effect.
Research Methods & Recommended Software
We use a variety of time-series analyses
for real-time (experimental) data, such
as phase-space analysis, recurrance
quantification, nonlinear regression,
entropy metrics, and symbolic dynamics
for pattern detection.

Nonlinear Dynamics, Psychology and Life Sciences
...is the quarterly research journal published
by SCTPLS. Refereed, highly rated
and widely indexed, NDPLS is an excellent
source of current progress and
classroom content. Authors are welcome
to submit manuscripts on all nonlinear
topics involving living systems.

Become a Member
SCTPLS has supported the development
of nonlinear science and its applications
worldwide through its publications and
conferences since 1991. Its members
are academics and practitioners who hail
from many walks of professional life.
Get entrenched, stay connected, live and
work on the cutting edge.
